Chemistry 2000, Section A
Spring 1996 Test 2 Solutions

1. 1. It sublimes.
   2. On the solid/liquid phase boundary, K=1. (Solid and liquid are in equilibrium and since the activities of pure solids and liquids are both 1, K=1.) In the portion of the phase diagram marked ``solid'', the solid, which is the reactant in the process described in this question, is favoured so we must have K<1.
   3. The liquid-vapour coexistence curve tells us the values of P and T at which water boils. We can see that this curve moves to larger values of T when P is raised. Therefore, the boiling temperature increases with pressure.

   Bonus:

   The triple occurs at a specific temperature. Therefore, if we observe all three phases, we know exactly what the temperature must be. This is different from the behaviour of sublimation, melting and boiling temperatures which all vary with pressure.

2. The temperature generally drops overnight. Vapour pressure decreases when the temperature is reduced. If the temperature drops enough, the partial pressure of water in the air becomes greater than the vapour pressure. The water vapour is no longer in equilibrium and it condenses. Condensation occurs preferentially on surfaces (like grass) acting as nucleators.
3. Here is my sketch:

   

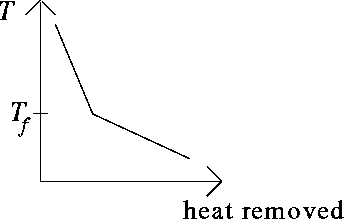
   In the sketch, is the freezing temperature of the solution. Since this is a solution, the solvent generally freezes out first. Once freezing starts, removal of heat thus causes some solvent to freeze, which leaves behind a more concentrated solution whose temperature can thus drop a little more.

4. We need the mole fraction of water so we need the number of moles of water and of the solutes: The number of moles of NaF is

   

   Since sodium fluoride dissociates in water, this represents 0.24mol of sodium ions and 0.24mol of fluoride ions.

   For the water, we use the density to calculate the mass:

   

   Using the molar mass, we then calculate the number of moles:

   

   The mole fraction of water is therefore

   

   Using Raoult's law, the vapour pressure is therefore

   

5. We want an osmotic pressure of 100Pa. We can immediately calculate the target concentration:

   

   We convert this concentration to mol/L:

   

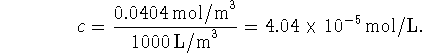
   This means that the number of moles of protein in our flask should be about

   

   If the molar mass of the protein is about 100000g/mol, we need

   

   of protein.

   Bonus:

   Proteins are often not very soluble so it's often not possible to make very concentrated solutions. (There are several other good reasons for using low concentrations.)