One must begin somewhere. Last spring the Mathematics Council of the Alberta Teachers' Association was meeting and we were all encouraged to think about making a presentation at his conference. I thought about it for a couple of minutes and came up with two titles:

• Number: Where Mathematics, Education and Children Meet.
• Tools and Notation: A Symbiotic Relationship for the Future.

The idea for the first presentation was to review some of the literature about Number, trying to bring together the three, often disparate, fields of mathematics, psychology and education. What is each group saying about number?

The idea for the second presentation was to discuss the tight relationship between how we express mathematical ideas and how this relates to various tools such as language, pencils, calculators, computers and the Internet.

The situation might be represented as follows:

The appropriate forms were filled out and submitted and months passed.

Then in late September we had another Mathematics Council meeting in Edmonton. As is my habit, I visited a couple of bookstores and bought a few books. One of these books was called Nexus, written by Mark Buchanan. The subtitle was "SMALL WORLDS and the Groundbreaking THEORY OF NETWORKS". This looked interesting. I had a vague idea about graph theory and networks but the phrase Small Worlds had no significance to me at the time. It looked interesting, had a few good reviewers comments on the back cover, a few diagrams and no equations. Should be light reading.

Another month passes ... I am aware that the conference is approaching and that I should begin making preparations for the two presentations. Avoiding this for the moment, I pick up Nexus and begin reading. I am immediately hooked. The next day I recommend it to a couple of colleagues as a "must read". A couple of days later we take the weekend off and drive to Great Falls, Montana for a little shopping. Waking up early, I finish reading Nexus. Later that morning we are in a bookstore in Great Falls. Browsing the math/science section I notice two books. One is called The Tipping Point and is mentioned in Nexus, the other is by Albert-Laszlo Barabasi and he is mentioned in Nexus. I buy them both. I also purchase a few other books.

Another night passes ... Very early on Saturday morning I begin making a network chart for a few of the books I bought the previous day.

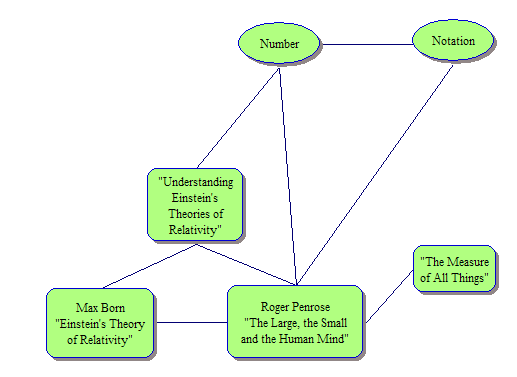
I had tried the first book, which I bought because of the word "understanding" in the title, but found it difficult. I then tried Max Born's book and found it very clear, at least at the outset. After an hour I opened up Roger Penrose's book. From the Preface, "... the work was not one I slaved over for years ... imperfection and sponteneity ...".

The penny drops. I will try to combine Penrose's perspective with that of Nexus to compose the two papers for the NCTM conference. Here is an image of my table at that moment:

My network diagram now looks like this:

So far, so good. At this point the paper splits into two parts. What follows is the Number paper. But as a parting image, here is a picture of the books near my home computer:

The stack with "The Number Sense" is for the Notation presentation and the tallest stack at the back is for the Number presentation. The book, "The Number Sense", is the latest arrival and should be on the stack at the back. You might consider this image as another form of network diagram. Now to bring order out of chaos.

First, a comment based on a cup of coffee with two colleagues at 8 this morning. At one point there was discussion about a workshop that one had attended a few days earlier. Basically it was viewed very positively. This then precipitated a few views on professional development. The question of a sequel to a successful workshop was raised. Our general sense was that there is seldom a sequel. For example, although there may be a handout which can be reviewed later, and the handout may include a series of references, it is rare that these are actually followed up, obtained, and thoughtfully read. The assumption appears to be that the event itself is adequate. Another form of sequel is a cup of coffee with a few colleagues where the content of the workshop is thoughtfully discussed. Also rare.

Number: Where Mathematics, Education and Children Meet.

As I begin to organize my resources, I scan my bookshelves and select those that appear to be most relevant. These are the books that I had placed on the floor. I then took those books and re-organized them on our kitchen table. I was able, without much effort, to place the 34 books in 6 piles, categorized according to some informal criterion of similarity. Let me begin by providing the typical list of References that one normally sees at the end of a paper or handout. Rather than use a standard citation format such as APA, I have opted to provide the author's full name, the year of publication, and the title, including a subtitle if there is one. This subtitle is often very useful in determining the content of the book. This is adequate information if one actually wanted to locate the book in a library, or order it from a bookstore or online supplier. The list is ordered by surname. This makes it easy to locate a reference if you already know the name of the author.

• Brainerd, Charles J. (1982). Children's Logical and Mathematical Cognition. Progress in Cognitive Development Research.
• Bruer, John T. (1993). Schools for Thought. A Science of Learning in the Classroom.
• Butterworth, Brian (1999). What Counts. How Every Brain is Hardwired for Math.
• Clawson: Calvin (1994). The Mathematical Traveler: Exploring the Grand History of Numbers. Perseus.
• Cohen, David K., McLaughlin, Milbrey W. & Talbert, Joan E. (1993). Teaching for Understanding. Challenges for Policy and Practice.
• Conway, John H. & Guy, Richard K. (1996). The Book of Numbers.
• Cooke, Heather (2003). Success with Mathematics.
• Davis, Robert B. (1984). Learning Mathematics: The Cognitive Science Approach to Mathematics Education.
• Debaene, Stanislas (1997). The Number Sense. How the Mind Creates Mathematics.
• Devlin, Keith (2000). The Math Gene. How Mathematical Thinking Evolved and Why Numbers are Like Gossip.
• Enzensberger, Hans Magnus (1997). The Number Devil. A Mathematical Adventure.
• Flannery, Sarah (2001). In Code. A Young Woman's Mathematical Journey.
• Flegg, Graham (1983). Numbers. Their History and Meaning.
• Forman, Ellice, A., Minick, Norris & Stone, C. Addison (1993). Contexts for Learning. Sociocultural Dynamics in Children's Development.
• Gelman, Rochel & Gallistel, C. R. (1986). The Child's Understanding of Number.
• Ginsburg, Herbert (1977). Children's Arithmetic: The Learning Process.
• Ginsburg, Herbert (1983). The Development of Mathematical Thinking.
• Gullberg, Jan (1997). Mathematics: From the Birth of Numbers.
• Hughes, Martin (1986). Children and Number. Difficulties in Learning Mathematics.
• Lakoff, George & Nunez, Rafael E. (2000). Where Mathematics Comes From. How the Embodied Mind Brings Mathematics into Being.
• Lesh, Richard & Landau, Marsha (1983). Acquisition of Mathematics Concepts and Processes.
• Liebeck, Pamela (1984). How Children Learn Mathematics: A Guide for Parents and Teachers.
• Ma, Liping (1999). Knowing and Teaching Elementary Mathematics.
• McLeod, Douglas B. & Adams, Verna M. (1989). Affect and Mathematical Problem Solving. A New Perspective.
• Newman, Denis, Griffin, Peg & Cole, Michael (1989). The Construction Zone: Working for Cognitive Change in School.
• Niederman, Derrick & Boyum, David (2003). What the Numbers Say. A Field Guide to Mastering Our Numerical World.
• Paulos, John Allen (1988). Innumeracy. Mathematical Illiteracy and its Consequences.
• Pickover, Clifford A. (2001). Wonders of Numbers. Adventures in Mathematics, Mind, and Meaning.
• Reys, Robert E., Suydam, Marilyn N. & Lindquist, Mary Montgomery (1989). Helping Children Learn Mathematics.
• Schoenfeld, Alan H. (1987). Cognitive Science and Mathematics Education.
• Seife, Charles (2000). Zero. The Biography of a Dangerous Idea.
• Smith. Karl J. (2004). The Nature of Mathematics. Tenth Edition.
• Wright, Robert J., Martland, Jim & Stafford, Ann K. (2000). Early Numeracy. Assessment for Teaching and Intervention.
• Wright, Robert J., Martland, Jim, Stafford, Ann K. & Stanger, Garry (2002). Teaching Number. Advancing Children's Skills and Strategies.

Here are an additional 8 books that are written specifically for elementary school teachers:

• Baroody, Arthur J. (1998). Fostering Children's Mathematical Power. An Investigative Approach to K-8 Mathematics Instruction.
• Bennett, Jr., Albert B. & Nelson, L. Ted (2001). Mathematics for Elementary Teachers. A Conceptual Approach. Fifth Edition.
• Bennett, Jr., Albert B. & Nelson, L. Ted (2001). Mathematics for Elementary Teachers. An Activity Approach. Fifth Edition.
• Billstein, Rick, Libeskind, S. & Lott, Johnny W. (2001). A Problem Solving Approach to Mathematics for Elementary School Teachers. Seventh Edition.
• Brumbaugh, Douglas, K., Rock, David, Brumbaugh, Linda S. & Rock, Michelle (2003). Teaching k-6 Mathematics.
• Kennedy, Leonard M., Tipps, Steve & Johnson, Art (2004). Guiding Children's Learning of Mathematics. Tenth Edition.
• Musser, Gary L., Burger, William F. & Peterson, Blake E. (2003). Mathematics for Elementary Teachers. A Contemporary Approach. Sixth Edition
• Van de Walle, John A. (2001). Elementary and Middle School Mathematics. Teaching Developmentally. Fourth Edition.

What can one do, or make, of such a list? It is almost overwhelming. Where is the wheat (beef, diamonds, ...)?

And this is just one source of information. There are a few other important sources that should not be overlooked:

• ERIC document retrieval system (formerly on microfiche, but now also available online as pdf files - assuming you are a registered user, i.e. a university student). This may be an area where professional organizations may be of assistance.
• Google. A popular search engine for the Web.
• Bookstores. Look for the math/science sections.
• Libraries, particularly university.
• Friends and colleagues. Priceless.

Returning to the list of 42 personal books, it is possible to group them into rough categories, and to construct a chart that shows this grouping. The chart is both complex and overly simple. The complexity arises from the number of nodes - it is difficult to comprehend 41 items all at once. It is even difficult when they have been "chunked" into seven clusters, with each cluster having less than ten nodes. The simplicity comes from the realization that only primary links have been shown. If all nodes were connected to each other there would be 41! links, a number that is about 10 raised to the power 38. And that assumes that there is only one idea per book. Fascinating! And these are only some of the books of one person, admittedly one who enjoys reading and playing with mathematics. This also gives us an insight into why there is so much debate about mathematics education. It is a very complex topic and all of the participants to the debate have widely and wildly different backgrounds.

As just indicated, the above analysis is highly biased toward the interests of one individual. Permit me to extend the bias by selecting a few important points from the resources displayed in the previous chart. First, let's get the primary categories separated from the detail:

• Mathematics
• Education
• Psychology
• Learning
• Research
• Student Centered
• For Teachers

My first assumption is that it is worthwhile to have some familiarity with each category. If one is teaching in the k-8 range, I would guess that the last category would be valued the highest. It is the most directly related to the daily activities of the classroom teacher. As you might expect, each of the books listed in this category is quite large. There is a substantial amount of information here.

Staying with my preferences, I would rate Mathematics as the next most important category. That is, one should have a fairly strong understanding of the actual mathematics that one is expected to teach. There is substantial research and documentation to suggest that this is often not the case. Without getting into the detail, a professional attitude would imply, I believe, a lifelong commitment to learning more about mathematics. There are many ways that might be approached, but the important issue is that it be approached, and approached continually. And this is only about the mathematics that is involved in understanding the various conceptions of number, and what one can do with numbers. Restricting myself to the books already listed, I would select the textbook by Smith and the delightful explorations of Conway as worth serious consideration.

There are three criteria that should be faced early, and made explicit, when one is composing a list of "desirabilities":

• conceptual appropriateness
• cost
• time

I have only mentioned conceptual appropriateness. As a general principle, I do not consider cost to be a critical concern. Even though the total cost can be quite substantial, one can spread this over time and according to other competitors for one's hard earned cash. That leaves time. Time is different than money. Although one normally thinks of time as infinite and money as finite, the opposite is more likely true. There are only so many hours in a day/week/month/year/lifetime. While money is not infinite in principle, we usually make adjustments so there is "enough" to meet our needs and at least some of our wants. When one begins buying books, one must be realistic about the amount of time one can devote to actually reading them. And one does not just read a good book, one studies it and thinks about it. Thinking takes time, as does drinking coffee.

So far, I would recommend one, two if I am feeling extravagant, of the books from the category of "For Teachers". I would then suggest the Smith book and the Conway book from the category of "Mathematics".

My third category, in order of personal importance, would be "Psychology". What do we presently know about how people learn and use numbers? Once again I find the choices difficult and find myself making further distinctions. Of the four books that appeal to me in this category, two are written for the public market and two are more specialized. I would pick one from each group. That is, one of {Debaene, Lakoff} and one of {Butterworth, Devlin}.

The emphasis now begins to shift toward the learner. Within the category of "Learning" I would select an older classic, Ginsburg's book on Children's Arithmetic. Then from "Student Centered" I would pick The Number Devil by Enzensberger.

From "Research" I would select two: Liping Ma's "Knowing and Teaching Elementary Mathematics" and Wright's "Teaching Number". Finally, from "Education" I would pick Bruer's "Schools for Thought".

This paper has not turned out as I expected. As I began I thought I would try to provide a synthesis of the literature on how the fields of Mathematics, Psychology and Education come together into one unified whole. Working my way through some of the books I have at hand, I have come to realize that would be unfair. The main beneficiary would be myself. That is not the intent of this presentation. The main benefit should accrue to the audience. In a very sincere way, as we all know as educators, one person cannot do the learning for another. For each of us, it is our minds that must change, as we continue to live and to learn.

I have indicated some of the thinking that I go through as I try to further my understanding of an area. I have shown how even my goals change as I become immersed in the topic. I have indicated my preference for developing visual images and diagrams to supplement and clarify my thinking. I am presently reading three books on network theory. I recommend all three to you as a new way of thinking about the relationships present in any large collection, and of how large collections can have the properties of a small world.

• Barabasi, Albert-Laszlo (2003) Linked. How Everything is Connected to Everything Else and What It Means for Business, Science and Everyday Life.
• Buchanan, Mark (2002). Nexus. Small Worlds and the Groundbreaking Theory of Networks.
• Gladwell, Malcolm (2000) The Tipping Point. How Little Things Can Make a Big DIfference.

I have also tried to show some of the difficulties implicit in being realistic - we all face boundary conditions. But overall I hope I have conveyed a sense of genuine excitement and involvement in mathematics education. I hope that the above discussion gives each of you a sense of the road map and of the many different routes available. I have indicated the paths I like, but I leave it to you to draw your own map, and to then pick your own vacation. Finally, if you see something that catches your attention, don't be afraid to change your plans and spend some time enjoying the unexpected.

Activity For Home Exploration

Use your Web browser to access your favorite search engine (I prefer Google since I have the Google toolbar added to my Internet Explorer layout). Type in the title of a book with quotes before and after, and the author's surname. Example:

"The Tipping Point" Gladwell

This will usually provide a few links, in addition to the one at amazon.com, to web pages that provide additional information about the book. These pages and reviews can be quite informative in their own right. It is a very useful way to spend a couple of hours, and to see which books demand your attention.

You may also try some keywords for content searches to find additional books that I do not have in my collection.

Learning to learn, which is an important component of professional development for any profession, now includes the judicious use of search engines! This applies not only to professionals but to learners of all ages. For example, try typing learn and fractions into a search engine, or Erdos. Professional Development comes in many guises. It includes both public and private activities. Public events include activities such as attending conferences and workshops, enrolling in a program of study for some form of additional credential or subscribing to a mailing list on the Internet. Private events may involve reading a book, using a search engine or simply surfing the web, accessing specific web based resources, developing a personal plan for professional development (why must everything be for credit?) or having a cup of coffee with colleagues to discuss, debate and argue a topic of interest. The sharing of viewpoints can be quite stimulating. Life-long learning is a life-style. It is a great hobby.

E-mail: dale.burnett@uleth.ca